Xah Math Blog Archive 2016-01
logician Raymond Smullyan is 97 now. ☹
Raymond Smullyan is famous among armature mathematicians for his many books on logic, presented as intriguing logic puzzles.
Knights and Knaves
On a island, all inhabitants are either knights, who always tell the truth, or knaves, who always lie.
John and Bill are residents of the island.
John says: We are both knaves.
Who is what?
here's his books Raymond Smullyan books
Why is Mandelbrot Set Cardioid Shaped?
more about cardioid here: Cardioid
[The Involute of a Cubical Parabola By John Baez. At https://johncarlosbaez.wordpress.com/2016/03/22/the-involute-of-a-cubical-parabola/ , accessed on 2016-05-14 ]
[The Capricornoid By John Baez. At https://johncarlosbaez.wordpress.com/2016/03/06/the-capricornoid/ , accessed on 2016-05-14 ]
Interactive Geometry of Plane Curves
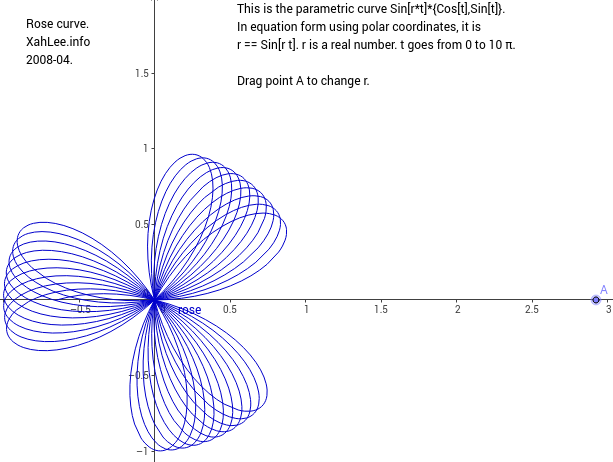
Plane Curves: GeoGebra Files Index
update complete. There are 60 of them. Enjoy!
Interactive Geometry of Plane Curves
following are interactive geometry of plane curves.
- 5-Point Conics
- Archimedean spiral
- Archimedes Circle
- Inversion of Archimedes's spiral
- Astroid Construction
- Astroid Construction
- Astroid's Pedal Curve
- Astroid Roll
- Astroid Trammel
They are GeoGebra software. Google no longer supports Java Applets. I'm updating them to html5 so they run in your browser. There are about 50 more to do, i'll be posting them in next few days. Hope you like them.
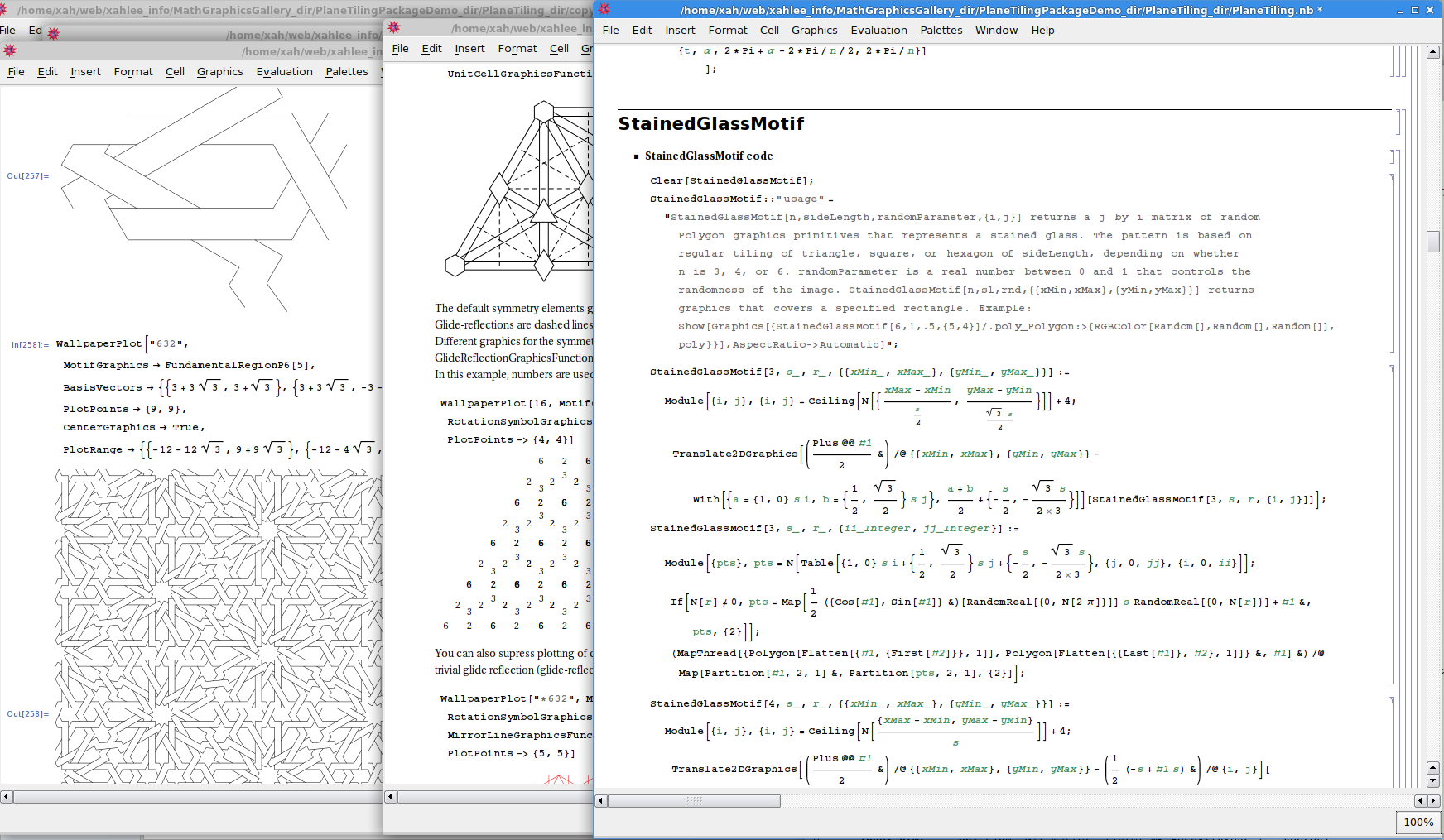
been working on WolframLang: Plane Tiling Mathematica Package
for illustration at the Nature of Associative Property of Algebra
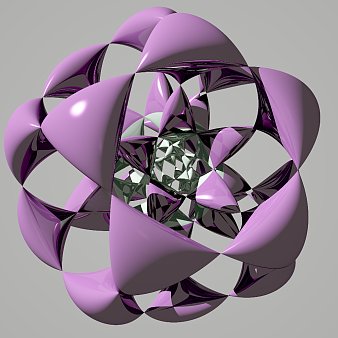
beautiful images of Barth Sextic
The Symmetries of Things by John H. Conway (Author), Heidi Burgiel (Author), Chaim Goodman-Strauss (Author) Buy at amazon
John H Conway's new old book
visual proofs is such that we have to artfully distribute the errors all over, so that in the limit, the error disappears!
Rectified Truncated Icosahedron and Elongated Square Gyrobicupola
John Baez again, from which i learned about the term “rectification” (of regular polyhedron), and also read what's archimedean solid, and learned about this strange beast Elongated square gyrobicupola .
the Elongated square gyrobicupola is interesting, besides the name, because
It is sometimes considered to be an Archimedean solid, because its faces consist of regular polygons that meet in the same pattern at each of its vertices. However, unlike the rest of the Archimedean solids, it lacks a set of global symmetries that take every vertex to every other vertex.
see Baez's article here: [Rectified Truncated Icosahedron By John Baez. At http://blogs.ams.org/visualinsight/2016/04/01/rectified_truncated_icosahedron/ , accessed on 2016-04-01 ]
by the way, i highly recommend this software KaleidoTile. See Great Math Software: Polyhedrons and Polytopes
Find Intersections of Lines. Bentley–Ottmann Algorithm
In computational geometry, the Bentley–Ottmann algorithm is a sweep line algorithm for listing all crossings in a set of line segments. Wikipedia Bentley–Ottmann algorithm
beautiful internactive demo (JavaScript) by Adam Pearce http://bl.ocks.org/1wheel/464141fe9b940153e636
Prime Number's Ending Digit, Probability
Soundararajan was drawn to study consecutive primes after hearing a lecture at Stanford by the mathematician Tadashi Tokieda, of the University of Cambridge, in which he mentioned a counterintuitive property of coin-tossing: If Alice tosses a coin until she sees a head followed by a tail, and Bob tosses a coin until he sees two heads in a row, then on average, Alice will require four tosses while Bob will require six tosses (try this at home!), even though head-tail and head-head have an equal chance of appearing after two coin tosses.
[Mathematicians Discover Prime Conspiracy. A previously unnoticed property of prime numbers seems to violate a longstanding assumption about how they behave. By Erica Klarreich. At https://www.quantamagazine.org/20160313-mathematicians-discover-prime-conspiracy/ , accessed on 2016-03-15 ]
see also
- [Biases between consecutive primes By Terence Tao. At https://terrytao.wordpress.com/2016/03/14/biases-between-consecutive-primes/ , accessed on 2016-03-15 ]
- [Unexpected biases in the distribution of consecutive primes By Robert J Lemke Oliver And Kannan Soundararajan. At http://arxiv.org/abs/1603.03720 , accessed on 2016-03-15 ]
Free Good Category Theory Book
Mathematician John Baez, and expert of category theory (and quantum mechanics), has a blog about it his course of category theory, at http://math.ucr.edu/home/baez/qg-winter2016/
in particular, he highly recommend this free book on category theory.
Emily Riehl, Category Theory in Context, 2014. Emily_Riehl__Category_Theory_in_Context_2014.pdf
Spare your arithmetic, never count the turns. Once, and a million!
—Shakespeare, in Cymbeline, Act II. Scene IV.
black holes and gravitational wave, by Stephen Wolfram

[Black Hole Tech? By Stephen Wolfram. At http://blog.stephenwolfram.com/2016/02/black-hole-tech/ , accessed on 2016-02-23 ]
enjoyed this article. If for nothing, go look at the 3-body problem animation.
classic textbook on algebraic geometry
This is the classic book on algebraic geometry.

though, i recognized the name. He wrote another book:

looking at amazon, apparently he wrote a few geometry texts. interesting is that he wrote one on Projective Geometry [Foundations of Projective Geometry Buy at amazon ]
You might find many good geometry texts at Plane Curves Books
… textbooks are too expensive. I think one can find lots of equivalents, cheap or free.
there's something wrong with pricing of textbooks in USA. The price tag is exorbitant. And i think the bottom line cause, is greed. No, am not inclined to blame anything or anyone just because they are successful or rich. But in the case of textbooks, i think indeed it's greed and schemes and made them so expensive, and universities have a lot to do with it.
educational institutions, such as universities, public or privately owned, as big organizations, they are not really doing the good they are supposed to do.
27 Lines on a Cubic Surface
[27 Lines on a Cubic Surface By John Baez. At http://blogs.ams.org/visualinsight/2016/02/15/27-lines-on-a-cubic-surface/ , accessed on 2016-02-17 ]
Visual Dictionary of Special Plane Curves now has side panel for easy navigation.
Google DeepMind AI, AlphaGo to Challenge World Champion?
you may have heard, that Google DeepMind AI, AlphaGo, is doing a human vs machine, challenge a go champion Lee Se-dol.
They said they beat European Champion 5-0, so is now challenging a champion.
I got excited, thought it happened sooner than i expected. Spent some 30 min to read about it. Turns out, that “European champion” is only ranked 2 dan. (Pros are 9 dan)
(go playing is ranked by “dan”, or “level” if you will. Rather a bad system. The chess elo system is much better. But basically, the “dan” is meant to be the number of moves a stronger player can yield to the other for a even game. That is, if you are 4 dan, and i'm 1 dan, that means, if you give me handicap 3 stones (i play 3 moves first, usually on designated spots), then we would have a “even” game. That's the ideal, anyway. In reality, there are professional ranks of dan and armature ranks of dan. Armature 9 dan is something like pro 5 dan.)
so, the AlphaGo human vs machine is rather marketing deception. Am very disappointed and annoyed by the marketing tactics.
this “news”, is rather no news. The go human champion would beat google's AI 5-0.


