Lissajous (in the works)
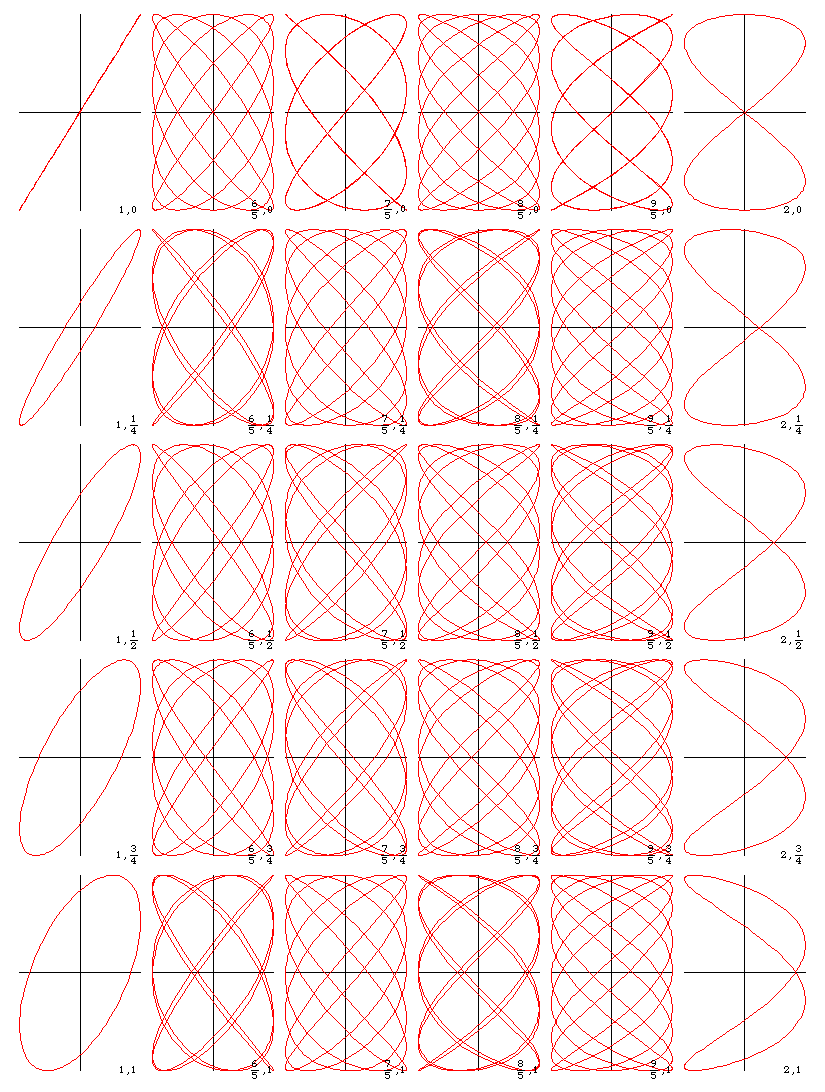
Description
Lissajous is a family of curves, given by {a*Sin[b*t+c], Sin[t]} with 3 parameters.
In studying nature there often arises the wave motion a*Sin[b*t+c]. For example, the motion of a pendulum. Lissajous is two such motions in perpendicular directions:
{a1*Sin[b1*t+c1],
a2*Sin[b2*t+c2]}
Changing the parameter by replacing t→1/b1*t then t→c2, then scale by 1/a2, we can simplify the parameters down to {a*Sin[b*t+c], Sin[t]}.
History
Studied by Nathaniel Bowditch in 1815 and Jules Antoine Lissajous (1822 to 1880).
Formula
Parametric: {a*Sin[b*t+c], Sin[t]}
The parameter a stretches the curve in one direction. Parameters b and c are more interesting. The period of the curve is the least common multiple of the two components, that is, LCM[2*Pi/b,2*Pi].