Chen Gackstatter

         About the Chen-Gackstatter Minimal Surface and its
                Analogs with Higher Dihedral Symmetry

                         H. Karcher

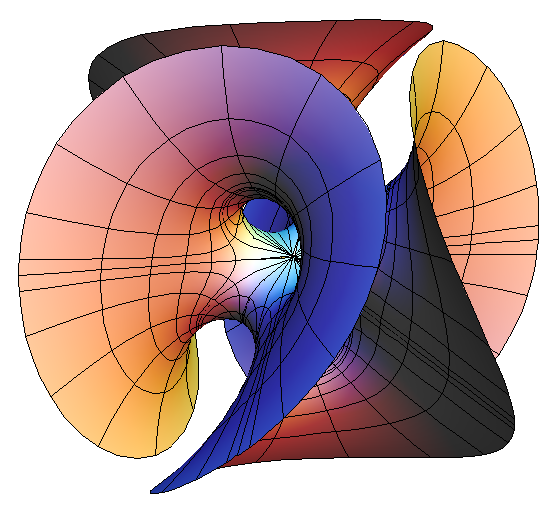
     This surface is the first finite total curvature immersion
of a Riemann surface of genus >0 (here the square Torus).

  It looks like an Enneper Surface with a handle added parallel to
its center saddle. This description determines the Gauss map
only up to a multiplicative constant (cc), which we took as the
morphing parameter. If this parameter is general then we get
a doubly periodic minimal immersion of the plane. The morphing
indicates how the period can be closed for one value of cc with the
intermediate value theorem.  The resemblance with the standard 
Enneper Surface is emphasized by using polar coordinates around 
the puncture. The dd=3 surface is an analogue which can be viewed 
as a higher order (120 degree symmetric) Enneper Surface with
a Y-shaped handle glued in. It was first published in a 1988 
Vieweg Calendar by Polthier and Wohlgemuth…..

See bibliography